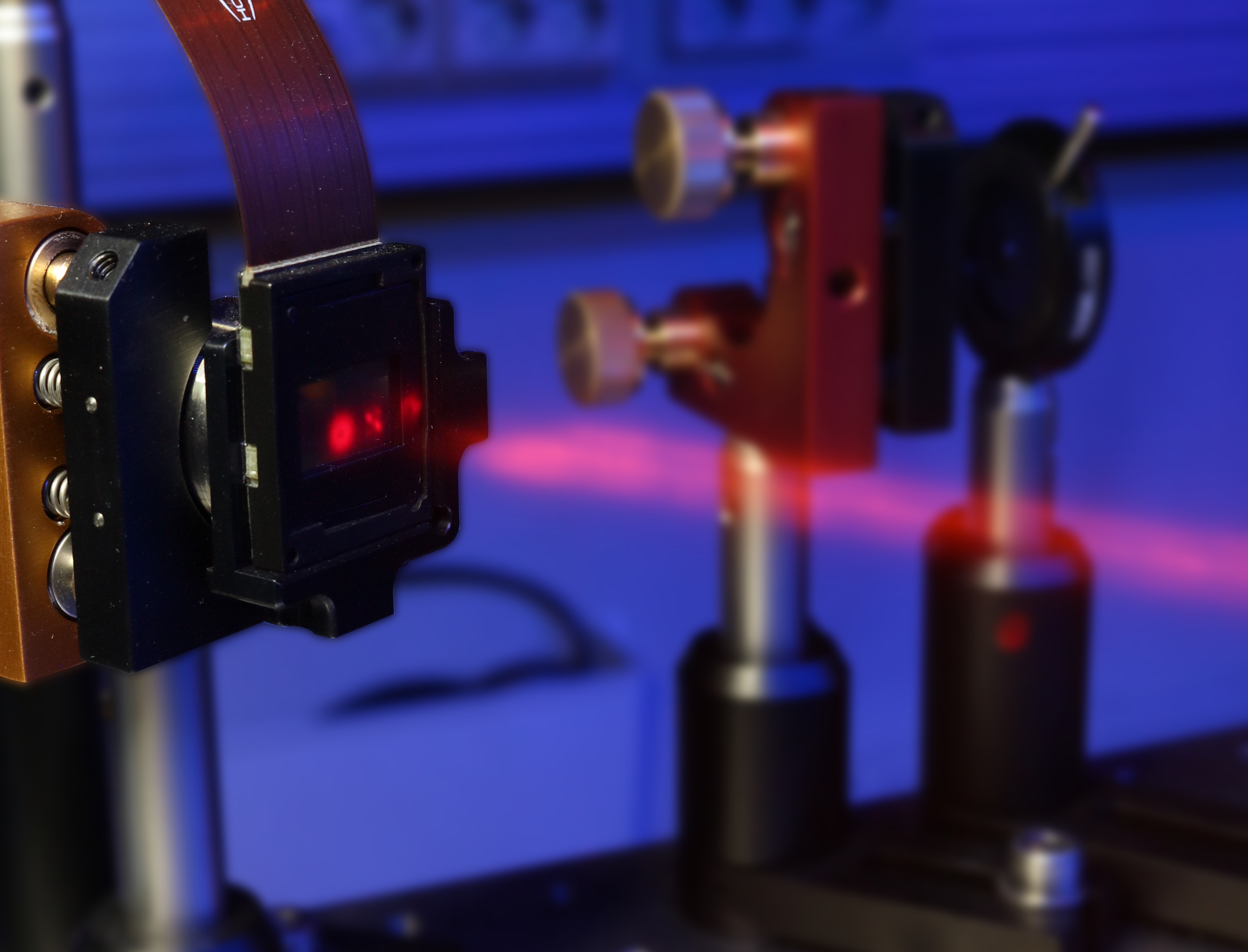
Markus Hiekkamäki, Tampere University
The Finnish Physical Society awards an annual prize for the excellent Master’s thesis in the field of physical sciences. The purpose of this prize is to support young researchers and acknowledge them for the exceptional work. The winner(s) are selected by the board based on the thesis work, references and articles/videos made by the finalists .
Markus Hiekkamäki received the shared first prize in Young Physicist 2019 competition. Below is the article he wrote about his thesis work.
Technologies relying on quantum phenomena have already revolutionized our way of life during the last century with devices such as transistors and lasers. Currently, these technologies are still being developed further and pushed to their limits within our increasingly fast computers and communication networks. Alongside them, a new research field has been growing since the end of the last century, which aims to harness quantum phenomena for revolutionizing the way we process information. This field is typically dubbed quantum information science and is most often fronted by the promise of a quantum computer that can outperform our classical computers in various useful tasks. The field of quantum information science also includes quantum communication, which offers the possibility of unconditionally secure communication protocols, in addition to quantum simulators that help us study physical systems. As can be seen from the many recent news articles, there is already a push for real world applications of these systems. A few examples are Google’s quantum computer and the satellite-based quantum communication system employed by Chinese research groups.
In any task of quantum information science, the reliable generation, manipulation, and measuring of quantum states is essential. This means that, regardless of the carrier we choose to hold this quantum information, we should be able to perform all the aforementioned tasks on the chosen degree of freedom of the carrier. For photons, the most common degree of freedom to use as a two-dimensional, i.e. binary, qubit state is polarization. This is because polarization already has a variety of devices that can be used to manipulate and detect it, e.g. waveplates and polarizing beamsplitters. However, over the last years it has been realized that having more than two possible bit values would be beneficial in most quantum information applications, especially in communication. One advantage would be the ability to transmit more information with a single photon when the chosen degree of freedom has more than two orthogonal states (e.g. horizontal and vertical polarization). We usually call these larger-than-binary quantum states quditsor d-dimensional quantum bits. The high-dimensionality offers a large alphabet of different values we can encode into our information carrier. With qudits, instead of just encoding values of 0, 1, or a superposition thereof, we can use values extending from zero to the limit of the dimensionality of our state space, along with the related superpositions. This larger alphabet also increases the robustness to errors in quantum communication protocols.
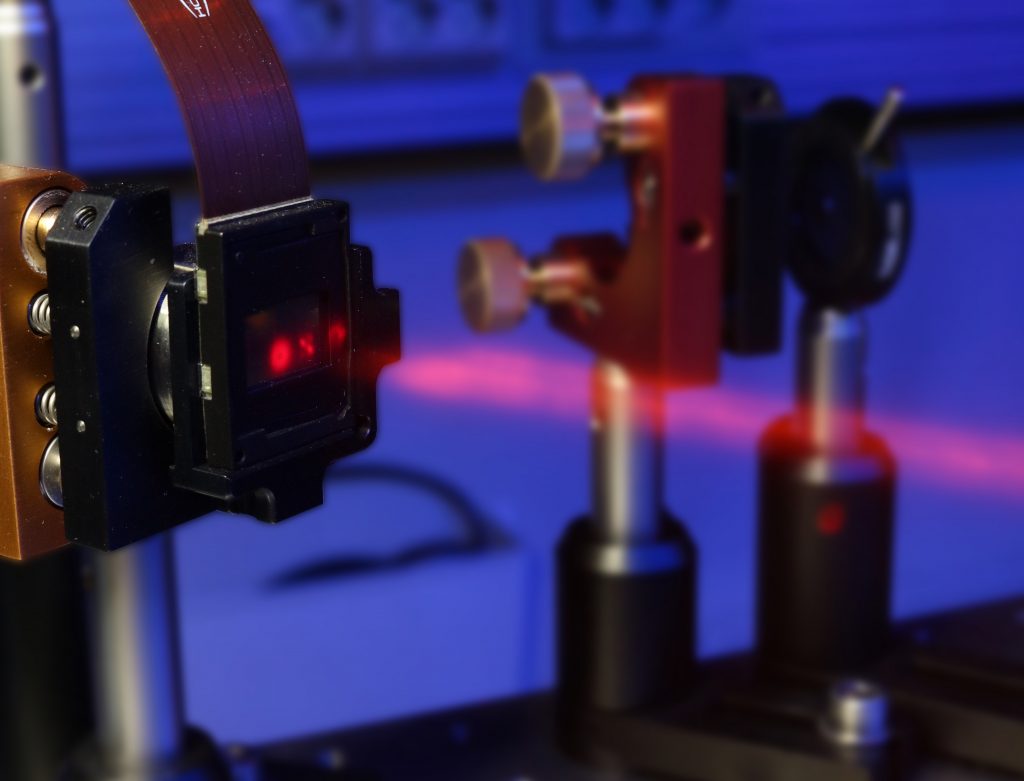
For photons, there are two paradigmatic degrees of freedom that can be used as our qudit state space, namely the temporal and spatial degrees of freedom. Both have their advantages, but our work is more focused on the spatial domain. However, in order to harness the spatial structure of photons for carrying bits of information, we first need to discretize this degree of freedom. Even though pixelating might be the most obvious discretization, we usually decompose the spatial structure of a light beams electric field into a set of orthogonal transverse spatial modes. These orthogonal structures we use are propagation invariant apart from some divergence during propagation. The mode structures contain two-dimensional phase and intensity information about the photon’s electric field on a plane perpendicular to its propagation direction.Furthermore, linear combinations, i.e. superpositions, of these structures can be used to describe any transverse structure of a collimated beam of light. This also makes the generation and detection of spatial mode superpositions easier in comparison to their temporal counterparts, since most of the same experimental methods apply for all spatial structures. Most importantly for us however, different orthogonal structures can be associated with a certain bit value, and since any complete set of spatial modes has an infinite selection of these orthogonal structures, we can ideally transmit any bit value from zero to infinity with a single structured photon. Naturally, the real-world restricts the usable state space with barriers such as finite apertures that are too small for more complex mode structures with larger diameters.
As already mentioned, in order to use these spatial photon structures for communication and computational tasks, we need to be able to control this degree of freedom for the photons. This would involve efficient methods for generating, detecting, and manipulating these transverse spatial modes. In the optimal case, the transformations applied in these tasks are completely unitaryin the chosen state space, meaning that no photon is sent into a mode outside of our chosen state space, e.g. through absorption or by errors in the experimental procedure. Hence, a method of generating and applying perfect, or near-perfect, unitaries on the photons would be highly beneficial. Additionally, if the method could be used for generating arbitrary transformations between different sets of modes, it could be used in implementing quantum gates, i.e. high-dimensional logic gates, for quantum computational applications of photonic spatial modes.
In my thesis, we implemented and tested a new method called wavefront matchingthat is a promisingly versatile tool for all the previously mentioned modulation task. Our goal was to test it in a few different experiments and gauge its potential for future investigations of complex multi-photon interactions. We started with testing transformations of a single-mode into a Gaussian beam mode, which is the intrinsic beam profile of a regular single-mode optical fiber. By utilizing this eigenmode of a single-mode fiber and our transformations, we built a spatial mode filter. The working principle stems from a single mode fiber only accepting photons with a specific spatial structure. By transforming one mode into this single spatial mode, i.e. a Gaussian, we filter out all photons with an orthogonal field structure. Compared to other mode filtering techniques, the major advantage of our filtering method are the significantly lower losses.
With this first experiment, we showed that wavefront matching can be used for spatial mode filtering and single-mode conversions. The next, more complex task was the implementation of multi-mode transformations, i.e. quantum gates. For spatial modes, these quantum gates are simply conversions from a set of input modes into set of output modes with each input mode having a well-defined output. One example of a high-dimensional quantum gate is a cyclic-NOT gate that is comparable to the NOT gate in classical computers. But instead of just changing the value 0 to 1 and vice versa, the high-dimensional cyclic-NOT gate needs to cyclically shift between a larger set of values. Furthermore, the gates also need to work with superposition states and entangled states, in order to be implemented in computational algorithms that take advantage of these quantum properties. For a truly unitary transformation these requirements are met. Hence, wavefront matching should be a great tool for constructing quantum gates, since it should produce unitary transformation.
In the second experiment, we implemented different quantum gates operating in 3-dimensional (bit-values 0, 1, 2) and 4-dimensional (bit-values 0, 1, 2, 3) state spaces. We tested different transformations with a laser beam as well as with single-photon states and achieved good error rates for a proof-of-principle experiment. Additionally, we confirmed the experimental unitarity of the transformations generated with wavefront matching, and thus we also confirmed their applicability in quantum computational tasks. We also implemented a unique local variation of a controlled-NOT gate on a single photon instead of the more common two-photon version needed for binary qubits. With this we hoped to showcase new possibilities the two independent quantum numbers of transverse spatial modes, along with their large alphabet, offer in computational tasks.
In the experiments we performed, wavefront matching displayed great potential for a multitude of different tasks, from generating and detecting structured photons to high-efficiency manipulation of these spatial structures. We hope that our work will trigger new advances in quantum communication, computation, and metrology with spatially structured single photons. Additionally, we hope to open new avenues for investigating novel multi-photon states and interactions with the complex transformations wavefront matching enables.
For more information
[1] Hiekkamäki, M. “Unitary transformations of spatial modes for quantum experiments”, Master’s thesis [2] Brandt, F., Hiekkamäki, M., Bouchard, F., Huber, M., & Fickler, R. “High-dimensional quantum gates using full-field spatial modes of photons.”Optica7.2 (2020): 98-107. [3] Hiekkamäki, M., Prabhakar, S., & Fickler, R. “Near-perfect measuring of full-field transverse-spatial modes of light.”, Optics express, 27.22 (2019): 31456-31464.